Your complimentary articles

You’ve read one of your four complimentary articles for this month.

You can read four articles free per month. To have complete access to the thousands of philosophy articles on this site, please

SUBSCRIBE NOW

Vagueness

Should We Take Vagueness Seriously?

Apostolos Syropoulos argues that vagueness is a virtue, sometimes.

Many descriptive words, such as ‘tall’, ‘beautiful’, ‘fat’, etc, etc, have something in common: they do not convey a precisely defined meaning. I assume you will agree with me that there is no general consensus on who is a tall person, who is a beautiful person, etc. Consider someone whose height is 170 cm (5ft 6in). Is this a tall person? If they’re male, we’re not really sure; but if the person is female, then she is definitely tall, particularly if she’s from a Mediterranean country. On the other hand, although we have learnt that the opposite of, say, ‘beautiful’ is ‘ugly’, still, that’s not the whole truth: There are millions of people out there that are not beautiful, but they’re not ugly either. They’re just ordinary people, like me, and probably like you.

Colours are quite interesting in this regard. People do not sense or appreciate colours in the same way. Some weird things happen as a result. I’m sure that you have experienced the following scenario: You’re standing in front of a beautiful and colourful landscape, and you decide to take several pictures of it. Later, you check these pictures on your computer or your phone, and you realize that the colours are not those you expected. Why not?

I’ve already hinted at an explanation of why this happens: colour is a subjective sensation. This means that we cannot directly or objectively measure or describe a colour. For instance, which one of the following colours are more red or less red than the others?

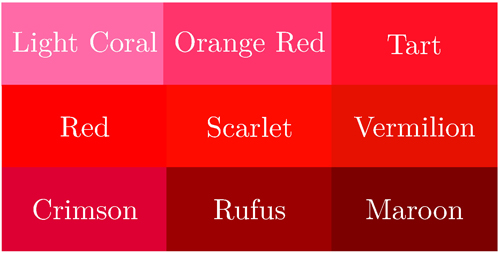
Some people may claim that red and scarlet are the same colour (trust me, they’re not). Others will claim that maroon is too dark to be red, while light coral is too light to be red. These and similar remarks show that we often cannot agree on things that we may have thought were very clear in our minds.

There are colour models – means by which we can describe colour comparisons in a predictable or systematic way. Still the problem remains that not all colours can be named or specified, since colours lie on a continuum. Also, the fact that there are many models means that there is no one unique way to describe a colour. Of course, these colour models are still quite useful, since they allow people to describe colours in a more precise way. But in a nutshell, for colour, height, and many other attributes, it is difficult to say that an object definitively has a particular property. The boundaries of the attributes are fuzzy, and depend on context, on our view of things, etc. This is why we cannot sometimes easily say whether something is light green or not, for example.

The ‘Sorites Paradox’, which was introduced by Eubulides of Miletus (fl. 4th century BC), is a famous argument that demonstrates fuzzy boundaries for concepts. The term ‘sorites’ (σωρείτες) derives from the Greek word ‘ soros’ (σωρός), which means ‘heap’, since the paradox was originally about how many grains of wheat make a heap. All agree that a single grain of wheat does not comprise a heap. The same applies for two grains. Or three. Or four… However, there is a point where the number of grains does become large enough to be called a heap – except, there is no general agreement when this occurs, and you might even be vague about it yourself.

A similar paradox is the ‘bald man’ paradox. Clearly, a man who has a full head of hair is not bald. If he loses one hair, he will still have a full head of hair. The same applies if he loses two, three, four, etc, hairs. However, if he loses enough hair he will become bald; and a man who has no hair at all clearly is bald – but there is no particular or specifiable number of hairs below which a man becomes bald.

In general, when we cannot be sure or agree whether someone or something has a particular property, we say that this property is vague. Some say that we see the person or object vaguely because we lack crucial information that prevents us from definitively categorizing that particular person or object. This is the epistemic view of vagueness. Others argue that the languages we speak have deficiencies which prevent us from properly judging whether a shirt is blue or not, or whether a person is tall or not. This is the semantic view of vagueness. However, there is a third view of vagueness, according to which objects, persons, animals, plants, etc, really are vague. This is the ontic view of vagueness.

Really Vague

In order to understand the ontic view of vagueness, let’s consider my dog Linda the pitbull. She is constantly losing hair, but at the same time new hair grows. Strictly speaking, Linda at 14:00 is not the same dog as Linda at 20:00, since during these six hours she will both lose and gain hair, may eat or poop, etc. A skeptical reader may object, claiming that she is the same dog, based on the idea that the dog is essentially the same. But if we say this, we also have to say that the individual dog really is a vaguely defined entity. And following this train of thought, we can also conclude that an old and a young person are the same person. Well, in one sense they may be, but in many senses they are not, since in general, people change. So they really are the same person, but only vaguely.

Let’s forget about dogs and hair, and think instead about geometrical objects, such as cubes and spheres. Everyone who has been taught some geometry knows the properties of these objects. For example, a cube has six faces, twelve edges, and eight vertices, and all edges have exactly the same length. Our question is: Are there cubes in the real world? Are there any such crisp (that is, non-vague) geometrical objects in the world we live in?

Perhaps surprisingly, the answer is that there are no cubes in the real world, although there are objects that are approximately cubes. Their edges have approximately the same length, and their faces are approximately parallel. More generally, in the real world there exist only approximations of pure abstract mathematically-defined objects.

As I hinted, not everyone believes that there really are vague objects. Indeed, in a short paper entitled ‘Can There Be Vague Objects?’ (Analysis 38: 1978), Gareth Evans ‘proved’ that there are no vague objects. He started by assuming that an object A is vague if there exists an another object B such that we cannot say whether ‘A = B’ is precisely true or false. Such statements of our vagueness can be called indeterminate. A simplified version of Evans’ proof then goes as follows:

1. The statement A = B is indeterminate.

2. From this we can conclude that B is indeterminately equal to A.

3. The statement A = A is determinate.

4. From this we conclude that A is not indeterminately equal to A.

5. From (2) and (4), we can determinately conclude that A is not equal to B.

The conclusion (5) apparently shows that there are no vague objects, since it shows that we can determinately know that A is not equal to B – which contradicts the idea that they are indeterminately (vaguely) equal. However, I think it is possible to show that even if Evans’ proof holds, this does not exclude the existence of vague objects. For example, there is an axiom in set theory that is called the axiom of extensionality. This axiom asserts that two sets are equal if they contain the same elements. iIn ‘On Evans’s Vague Object from Set Theoretic Viewpoint’ (Journal of Philosophical Logic 35:4, 2006), Shunsuke Yatabe and Hiroyuki Inaoka proposed that A is a vague object if and only if the axiom of extensionality does not hold for A. The interesting things is that the axiom of extensionality has no real importance for the formalization of mathematics. This means that we can have vague objects in a mathematical universe where at the same time Evans' proof holds. Of course, we could refute the proof by noting that it reasons about vague objects in a setting where only two truth values are admitted, but this a quite an elaborate task.

Inexact Science

Our discussion of vagueness so far makes me ask myself this: If vagueness is the norm, then why does science apparently ignore this important fact?

It seems that people have a deep respect for objects with exact properties, and that anything otherwise makes us feel uncomfortable. According to the following excerpt from ‘The Life of Marcellus’, which is part of Plutarch’s Parallel Lives (c.100 AD), we see that Plato was against the study of curves that could be drawn only with the help of ‘mechanical devices’ for precisely this reason:

“For the art of mechanics, now so celebrated and admired, was first originated by Eudoxus and Archytas, who embellished geometry with its subtleties, and gave to problems incapable of proof by word and diagram, a support derived from mechanical illustrations that were patent to the senses… But Plato was incensed at this, and inveighed against them as corrupters and destroyers of the pure excellence of geometry, which thus turned her back upon the incorporeal things of abstract thought and descended to the things of sense, making use, moreover, of objects which required much mean and manual labour. For this reason mechanics was made entirely distinct from geometry, and being for a long time ignored by philosophers, came to be regarded as one of the military arts.”

In the physical sciences, vagueness sometimes turns up in the most unexpected places, such as the world of computing. When engineers were building the first computers, they needed to find a way to represent information. Encoding dozens of symbols, or characters, is difficult, because one needs many distinct physical states to represent each character. However, it is possible to represent each character by a number through a ‘mapping’, which simply means that we can construct a table where characters are mapped to consecutive integers: ‘a’ → 1, ‘b’ → 2, etc. Since the decimal numbering system uses ten digits to encode numbers, we would need ten distinct physical states to represent these ten digits. But the decimal numbering system isn’t the only one. The simplest is the binary system, which has only two digits, ‘0’ and ‘1’. Encoding two digits in an physical system is relatively easy, and one can exploit electric currents to represent these two digits. Thus the people who built early computers decided that when a current travels along a wire, we should assume that the digit ‘1’ has just travelled along the wire; similarly, when no current travels along a wire, we should assume that the digit ‘0’ has just traveled along the wire.

But how do we detect if a current travels along a wire or not? We measure the potential difference between two parts of the circuit. More specifically, if the potential difference at a given part of the circuit was, say, 3.5 V, then the computer pioneers assumed that the digit ‘1’ had just passed through this part. If the potential at the same part of the circuit was, say, only 0.3 volts, then they assumed that the digit ‘0’ had just passed through it. The problem here is that the potential is not always exactly 3.5 Volts or 0.3 Volts. Voltage fluctuations happen, perhaps because there are loose or corroded connections either at base or on the powerlines, or because of bad weather, or extreme heat, and so on. Indeed, there are many things that can cause voltage fluctuations, and so the measurements are always approximate. In a nutshell, although computers are supposed to be precise devices, they operate on imprecise power sources and differences and thus they are vague by nature. Naturally, the computer pioneers wanted to be able to compute precise quantities, and so they did everything to get rid of the extra feature of the power being measured, its inexactitude. Yet, as various more modern approaches to computing have revealed, it is possible to incorporate vagueness to get better results, with systems that operate more smoothly than their counterparts which completely ignore vagueness.

Vagueness is an intrinsic part of quantum mechanics, the study of the world at the atomic and subatomic level. In particular, Heisenberg’s Uncertainty Principle tells us that we cannot know exactly both a wave-particle’s position and its momentum (or other given pairs of properties). We must choose to ignore these features if we want to talk in exact terms. Moreover, at school we learnt that atoms have a nucleus consisting of little spheres – protons and neutrons – with other little spheres – electrons – orbiting it. But this is an oversimplified, if not totally wrong, picture of the atom. In fact, each electron, proton, or neutron is more like a cloud of probabilities, and we have no way to exactly predict its position. Thus the position of an electron around an atom is a vague concept. And perhaps I ought ot add that electrons, and indeed elementary particles more generally, are not spheres, but rather, their shapes are indefinite.

We are similarly taught that planets, moons, and stars are only imperfect spheres. Thus the laws of physics that describe the motions of these objects are necessarily approximate (that is, vague), since we are not dealing with geometrically perfect objects. But, someone may ask, if nothing in astrophysics is precise, then how do we manage to send probes to distant planets, moons, and asteroids, and get important information about these worlds?

First, one must remember that there have been many failures, and many probes have failed miserably – by crashing, or perhaps by disappearing completely. On the other hand, many probes have successfully completed their tasks. However, when compared to a comet, or even a little ex-planet like Pluto, a probe is really a very small object. Thus we can accurately enough calculate the trajectory of a probe by making a number of assumptions. If everything goes well (that is, if we are lucky), the probe will reach its destination safe and sound. But, as should by now be obvious, here we’re not talking about an ideal science where everything is smooth and there are no unexpected events or phenomena. Real, honest science assumes that the world is vague, imprecise, and unpredictable.

In 2002 Daniel Kahneman shared the Nobel Prize in Economic Sciences with Vernon L. Smith. In 2016, the Harvard Business Review published a compelling article coauthored by Kahneman, Andrew M. Rosenfield, Linnea Gandhi and Tom Blaser, in which the authors noted that “Humans are unreliable decision makers; their judgments are strongly influenced by irrelevant factors, such as their current mood, the time since their last meal, and the weather. We call the chance variability of judgments noise.” What Kahneman and his co-authors here call ‘noise’ is surely another manifestation of vagueness. Kahneman used this to put forth the idea that the errors that happen during information propagation are caused by the vagueness-inducing environment where information flows.

Because of the COVID-19 pandemic, many people got to know a lot about viruses, bacteria and all these tiny living things. What was a big surprise for most laypeople is that the scientific community is divided on whether viruses are indeed living things. In fact, it seems we can say that viruses are living things ‘to some degree’. This automatically classifies them as vague entities. Vagueness is prevalent throughout the life sciences, and even the definition of a ‘species’ is a manifestation of this. Typically, a species is assumed to be the largest group of organisms that have common characteristics and are capable of interbreeding. But this vague definition can lead even a non-biologist to conclude that it is quite possible that some individuals might at the same time both be members and not members of some species. Are Neanderthals and Homo sapiens the same species? We say they’re different; but the Neanderthals interbred with humans a lot in the past. So species is yet another vague scientific concept.

If vagueness goes down to the level of viruses, where chemistry plays an important role, then naturally our next question is whether chemistry itself is vague? Prebiotic chemistry is the study of the chemical steps that lead to the first living organisms. One big problem here is that (as we have seen with viruses) there is no unambiguous and universally accepted definition of ‘living organism’.

Of course, all this vagueness does not stop doctors or biologists from doing excellent work. Similarly, physicists may not fully understand the nature of time or space, but this does not prevent them from making discoveries in physics.

There are many other examples of vagueness in science, but I simply wanted to show that vagueness exists in science and that in many cases it is ignored. I think this is a rather unfortunate practice. We should admit vagueness in order to better understand how things work in real-life situations.

Apostolos Syropoulos is an independent scholar working in computability theory, vagueness (fuzzy mathematics, computation, etc) and digital typography. He lives and works in Xanthi, Greece.