Your complimentary articles

You’ve read one of your four complimentary articles for this month.

You can read four articles free per month. To have complete access to the thousands of philosophy articles on this site, please

SUBSCRIBE NOW

Philosophical Science

Mirror, Mirror

Tim Wilkinson reflects on an old question.

Why do mirrors reverse left with right but not up with down?

It’s a problem with a long history. Plato addressed it in his dialogue Timaeus in the Fourth Century BCE, but was somewhat hampered by the prevailing theory of vision. Following Empedocles, Plato thought sight is explained by rays emitted from fire in the eyes. To account for the fact that we can’t see at night, Empedocles suggested that there must be some interaction between the rays emanating from the eyes and those emitted by other fires, such as the sun. The theory that sight is the result of an external influence entering the eyes was proposed by Aristotle somewhat later. Even making allowances for his defective theory of vision, Plato’s explanation of left-right inversion in a mirror is not particularly convincing: “The fires from within and from without meet about the smooth and bright surface of the mirror; and because they meet in a manner contrary to the usual mode, the right and left sides of the object are transposed.” (Timaeus). To improve on Plato’s explanation, we need to unpack the notion of left-right reversal, and make it clear what plane (flat) mirrors (henceforth just ‘mirrors’) do, and just as importantly, what they don’t do.

Mirror-Speak

The problem is partly one of ambiguous terminology. There are several possible meanings of ‘left-right’, only one of which is the same kind of concept as ‘up-down’. This can easily be seen by facing a mirror and reaching out to touch the glass with one hand. It will then be obvious that the reflection of your right hand is directly opposite your right hand rather than your left, the reflection of your left hand is directly opposite your left hand rather than your right, and, as expected, the reflection of your head is opposite your head, rather than, say, opposite your feet. Similarly, if you face a mirror with a ball in one hand and a racquet in the other, the ball does not have the racquet as its reflection. Therefore, in the same sense that mirrors don’t swap up with down, they don’t swap left with right either. Left-right mirror inversion is in relation to a meaning of ‘left-right’ that’s a completely different kind of concept from ‘up-down’ inversion, as will hopefully become clear.

But wait! Place a mirror face up on the ground, and stand upon it. Now the mirror does reverse up and down. Cruel shiny thing! Why do you mock us so? In fact, I shall show that there is no inconsistency here either, other than the inconsistent way we are using ‘up’ and ‘down’.

The Mirror Rule

In all the situations above, the same property of mirrors is at work: Every point and its reflection are directly opposite each other (a line drawn between the two is always perpendicular to the mirror) and lie at the same distance from the mirror plane. Let’s call this the ‘mirror rule’. It turns out that the mirror rule can be deduced from an even more basic fact we all learned at school, namely that when light meets a mirror, its angle of incidence equals its angle of reflection. Behind the laws of reflection lies Nobel laureate Richard Feynman’s still deeper explanation in terms of quantum theory. For our purposes, however, it is easier to work with the mirror rule.

Most people do not find the mirror rule at all paradoxical or confusing. Notice that it is completely independent of notions of left-right and up-down, in the sense that it applies at all times, to all objects, irrespective of how they are oriented with respect to the observer, the mirror, and each other.

Left & Right

Having established that mirrors treat ‘left-right’ and ‘up-down’ equally in one sense (in which they do not reverse anything at all), we need to unravel what people mean when they say that mirrors reverse left and right.

There is a reasonably large body of work on the subject. Martin Gardner gave an excellent account in The New Ambidextrous Universe (1964), which triggered a cascade of papers in philosophy journals, including Ned Block’s ‘Why Do Mirrors Reverse Right/Left but Not Up/Down?’ (Journal of Philosophy, Vol. 71, 1974), and Don Locke’s ‘Through the Looking Glass’ (Philosophical Review, LXXXVI, 1977). To my mind what’s remarkable about these papers, when their accounts are broadly consistent, is the lengths to which their authors are prepared to go to disagree with each other, and especially with Gardner. For example, Block introduces no less than four separate meanings of ‘left-right inversion’ (one, for example, is the sense in which writing appears to be inverted, and another the sense in which if I point to my right, my mirror image points to his left), and alludes to several more, before claiming that Gardner has failed to explain them all. However, on a more sympathetic reading of both accounts, Block’s four types of inversion are special cases of what is already explained by Gardner. Rather than try to reconcile the various treatments I shall give my own version of the solution, based loosely on Gardner’s, which I wholeheartedly endorse.

Mirrors & Consistency

Hand pictures © Tim Wilkinson 2016

At least as far back as ancient Greece it has been generally accepted that whatever the universe is like, it must be consistent. It follows that any mirror that obeys the mirror rule cannot perform any optical trickery inconsistent with that rule. But since the mirror rule doesn’t mention left, right, up, or down, how can left-right inversion possibly be deduced from it?

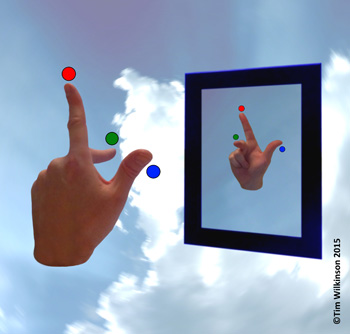
At this point, it’s necessary to make a brief diversion into geometry. Lest I be accused of cheating by bringing mathematics into a philosophical debate, I’ll pre-emptively let one of the founders of modern logic, Gottlob Frege, state the case for the defence: “A philosopher who has nothing to do with geometry is only half a philosopher, and a mathematician with no element of philosophy in him is only half a mathematician. These disciplines have estranged themselves from one another to the detriment of both.” (Gottlob Frege: Posthumous Writings, ed Hermes et al, 1979). I’ll have more to say about the interdisciplinary lessons to be learned from the mirror problem later on, but for now let’s examine the geometrical argument, which at least has the virtue of being short. Consider the following hand and its reflection:

Notice that the shape of the reflection is uniquely and unambiguously determined by the mirror rule. In other words, given the mirror rule, the shape of the reflection could not be anything other than it is. Now consider the two hand shapes. Are they the same? Clearly not. The reflections indicated by the red and blue dots more or less line up with the originals, but the green finger is pointing in completely the wrong direction.

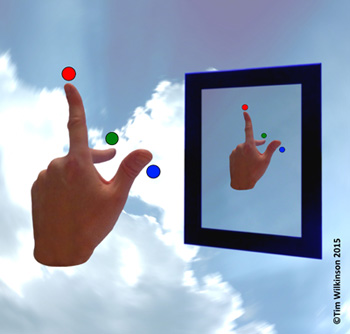
Conversely, consider the following diagram in which the hand and its erstwhile reflection are the same shape:

This new ‘reflection’ can nearly be oriented so that its red and blue fingers obey the mirror rule at the same time, but the mirror rule is completely broken for the green finger, whose reflection is too far away from the mirror. In short, consistency demands that if the mirror rule is obeyed, the original and reflection hand shapes cannot be the same.

It is natural at this point to wonder why mirrors obey the mirror rule. It can be deduced from some even more basic facts about optics (or from quantum theory if you prefer), but that’s almost beside the point. There could well be other universes, or (though I seriously doubt it) even other parts of this one where mirrors do not obey the mirror rule. But there is no possible world in which shiny surfaces observe the mirror rule and an asymmetrical object like a hand is the same shape as its own reflection, since such a world would be self-contradictory. Certain objects, such as cubes, do have the same shape as their reflections; but even then, unless the shape happens to be in one of a few special positions with respect to the mirror, or even more symmetrical than a cube, as in the case of a billiard ball, mirrors usually alter orientation. Again, these facts are direct and inevitable consequences of the mirror rule.

Hands & Handedness

Objects that cannot be directly superimposed on their own reflection are called chiral, and a chiral object and its mirror image are called enantiomers. Such concepts are extremely important in chemistry, where they are used in connection with the shapes of molecules. The room in which I am sitting is chiral, as is my house, and the town where I live.

The word ‘chiral’ derives from the Greek word for ‘hand’, because our two hands are more or less mirror images of each other. Most animals exhibit something close to bilateral symmetry of this kind (there are exceptions, such as snails and the male fiddler crab). Even our own bodies are asymmetric in certain respects, including the internal placement of organs such as the heart. Why we are nearly symmetric is a biological question rather than a philosophical or geometrical one, but given that it is undeniably the case, we are finally able to examine the way in which mirrors invert left and right.

‘Righty’, the famous male fiddler crab
Fiddler crab © Andrea Westmoreland 2016

Although each of my hands is chiral, my whole body is not, at least approximately. Therefore, when I examine my reflection in a mirror, I see another properly formed person. The reflection of my head is opposite my head, and the reflection of my right hand is opposite my right hand. However, it is not a right hand. In fact, it will be the same shape as my left hand – but that’s a biological accident. Writing in the mirror world looks extremely strange. Writing is chiral if written on paper, but interestingly, not chiral if written on a transparent substance such as clear plastic and held up to the mirror, as the reader can easily verify. When I look in a mirror, the world I see is superficially similar to ours, but is its chiral twin. It has to be so, for consistency with the mirror rule. If I raise my right arm, my mirror image raises the image of my right arm, but in shape it’s my mirror-twin’s left. All these effects are manifestations of the same thing. Since my reflection looks altogether human, it is natural for me to imagine myself in his place, embedded in a mirror world where someone seems to have rearranged my furniture. When we say that mirrors reverse left with right, we are not making a statement about direction. If I face a mirror and point to my right, my mirror twin points in the same absolute direction I do. It is only if I imagine myself inhabiting his body that he appears to be pointing left rather than right.

When I stand on a mirror placed on the ground, the mirror rule demands that the reflection of my feet be close to the mirror and my reflected head further away, and so ‘up’ becomes ‘down’ because the mirror happens to be on the floor. For the same reason, if I stand sideways-on rather than face-on to a mirror, and point to my right, my alter ego now does point the opposite way. But in each case, the mirror reverses only the direction at right angles to its surface, as the mirror rule demands that it must. ‘Up’, ‘down’, ‘behind’ and similar concepts are relative to the observer. ‘Left’ and ‘right’ are also relative to the observer in the sense of the observer’s direction, but absolute in the sense that my right hand does not become a left simply because I turn around or stand on my head. So in the directional sense, mirrors do sometimes reverse ‘up with down’ or ‘left with right’ and sometimes not, in strict accordance with the mirror rule, depending on how the mirror, the Earth, and the observer are situated with respect to one another. In contrast, mirrors always reverse left with right in the sense of geometry, because the mirror rule demands that the reflection of my right hand must always be a left hand for the person in the mirror. So when we speak of ‘left-right inversion’, we are in fact imagining ourselves transported into the mirror world and trying to make sense of what we find there.

Mirrors obey the mirror rule, and as a consequence they unavoidably alter the geometry of the world and of most shapes within. They also usually alter orientation. That’s what mirrors do, and that’s all that they do. For as long as the mirror rule is respected, no other outcome is possible in a consistent universe.

Philosophy & Science

The philosopher Stephen Law has cited left-right inversion in a mirror as an example of a problem science cannot solve, where “only philosophy will do” (Think Vol 11, No.30). In a live debate in Oxford in 2013, Law put this suggestion to Richard Dawkins, whose response was that the mirror puzzle is actually a scientific problem, and a trivial one at that.

In the real world of education and job applications, dividing our knowledge into subject areas is unavoidable and reasonably helpful, and some wrangling over where the borders lie is to be expected. But it is worrying to see how rapidly such exchanges can degenerate on the internet or in print. Before you know where you are, otherwise sensible academics are pronouncing that philosophy is a waste of time. (For those interested in such altercations and in an analysis of where things go wrong, Massimo Pigliucci’s website and blogs are good places to start.) When Stephen Law says that only philosophy can solve the mirror problem, in part he is making the wider point that science is not the only legitimate source of knowledge. On that score I couldn’t agree more. But a proper comprehension of mirrors, and much else besides, can equally not be arrived at by philosophy alone. The answer is partly scientific – the mirror rule and its underlying physics – partly mathematical/geometrical, and partly philosophical – the careful unpicking of concepts such as ‘left’, ‘right’, ‘up’ and ‘down’ and their different uses or senses. The artificial barriers we have erected between disciplines may have sprung up along natural fault lines, and may sometimes be helpful, but they should be enthusiastically torn down the moment they begin to impede understanding.

A Final Twist

Earlier I argued that the reflection of a right hand is a left hand, and that the one cannot be simply superimposed on the other. This statement relies on some hidden, if reasonable, assumptions about the nature of space itself.

Consider the Möbius strip, which is formed by taking a length of paper and, after introducing a single twist, joining the ends together. Now, by rotating a left hand and taking it on a journey around the strip, it can be transposed into a right hand without the use of a mirror:

What about the geometry of the physical universe? Is it like a Möbius strip?

At human scales space definitely appears to be flat. Recent measurements of the cosmic microwave background radiation strongly suggest that it is also flat over the largest observable distances (albeit with some curvature at intermediate scales, due to the influence of gravity). If so, space is not closed as a Möbius strip is, and no matter how far you travel in a straight line, you will never return to your point of origin, reflected or not.

Back on Earth, science is the best way thus far devised of studying the regularities and composition of the universe; but it is far from being the only reliable source of human knowledge. I’m not only thinking of mathematics and logic. We can contemplate a host of ethical and other crucially important problems which science may inform, but that it can never solve alone. As I hope the mirror puzzle shows, and as Frege wisely counselled, we need to guard against our methods of enquiry becoming dysfunctionally estranged from each other. If we can resist our innate tendency to look down at one another and instead turn our gaze skywards, we may be rewarded with a glimpse of astonishing truths far more mysterious than any mirror.

Tim Wilkinson used to teach mathematics at The University of Newcastle-Upon-Tyne. He hopes a truce will soon be announced in the conflict between science and philosophy.